Properties of the derivative solved exercises

Basic properties of the derivative

The basic properties of the derivative to find the derivative of a function are:

Derivative of a constant function

If \(f\) is a constant function, that is, \(f(x)=k\), then:

\[f’(x)=0\]

This property states that the derivative of a constant function is equal to zero.

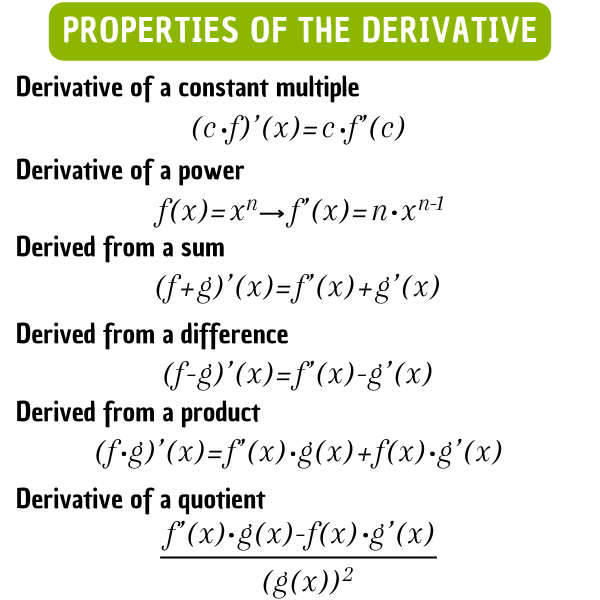
Derivative of a constant multiple

If \(f\) is a function differentiable on \(x\), and \(c\) is a constant, then:

\[\left(c\cdot f\right)’(x)=c\cdot f’(x)\]

This property states that the derivative of the product of a constant and a function is equal to the constant multiplied by the derivative of the function.

Derivative of a power

If \(f\) is a function defined by \(f(x)=x^n\), where \(n\) is any real number other than zero, then:

\[f’(x)=nx^{n-1}\]

This property states that the derivative of \(x\) raised to any exponent is equal to the exponent multiplied by \(x\) raised to the same exponent minus one.

Recommended article: Properties of powers solved exercises

Derivative of a sum of functions

If \(f\) and \(g\) are differentiable functions on \(x\), then:

\[\left(f+g\right)’(x)=f’(x)+g’(x)\]

This property states that the derivative of a sum of functions is equal to the sum of the derivatives of each of the functions separately.

Derived from a difference of functions

If \(f\) and \(g\) are differentiable functions on \(x\), then:

\[\left(f-g\right)’(x)=f’(x)-g’(x)\]

This property states that the derivative of a difference of functions is equal to the difference of the derivatives of each of the functions separately.

Derived from a product of functions

If \(f\) and \(g\) are differentiable functions on \(x\), then:

\[\left(f\cdot g\right)'(x)=f'(x)g(x)+f(x)g'(x)\]

This property states that the derivative of the product of two functions is equal to the sum of the product of the derivative of the first function by the second, plus the product of the first function by the derivative of the second function. In other words, the derivative of the product of two functions is equal to the derivative of the first function multiplied by the second function unchanged, plus the first function multiplied by the derivative of the second function.

Derivative of a quotient of functions

If \(f\) and \(g\) are differentiable functions on \(x\), then:

\[\left(\frac{f}{g}\right)'(x)=\frac{f'(x)g(x)-g'(x)f(x)}{\left[g(x)\right]^2}\]

This property states that the derivative of a division of two functions is equal to the derivative of the numerator function multiplied by the denominator function, minus the numerator function multiplied by the derivative of the denominator function, all divided by the square of the denominator function.

Derivatives solved exercises

Exercise 1. Find the derivative of the function \(f\) defined as: \[f(x)=x^3+x^2\] Solution: To find the derivative of the function we apply the following properties of the derivative:

\[\begin{aligned}f’(x)&=\frac{d}{dx}f(x)\\&=\frac{d}{dx}\left(x^3+x^2\right)\\&=\frac{d}{dx}x^3+\frac{d}{dx}x^2\quad\text{Property of the derivative of a sum}\\&=3x^{3-1}+2x^{2-1}\quad\text{Property of the derivative of }x^n\\&=3x^2+2x\end{aligned}\]

Therefore, the derivative of \(f\) is \(f'(x)=3x^2+2x\).

Exercise 2. Find the derivative of the function \(f\) defined as: \[f(x)=x^8-x^4\] Solution: To find the derivative of the function we apply the following properties of the derivative:

\[\begin{aligned}f’(x)&=\frac{d}{dx}f(x)\\&=\frac{d}{dx}\left(x^8-x^4\right)\\&=\frac{d}{dx}x^8-\frac{d}{dx}x^4\quad\text{Property of the derivative of a difference}\\&=8x^{8-1}-4x^{4-1}\quad\text{Property of the derivative of }x^n\\&=8x^7-4x^3\end{aligned}\]

Therefore, the derivative of \(f\) is \(f'(x)=8x^7-4x^3\).

Exercise 3. Find the derivative of the function \(f\) defined as: \[f(x)=x^5+8\] Solution: Applying the properties of the derivative, we obtain:

\[\begin{aligned}f’(x)&=\frac{d}{dx}f(x)\\&=\frac{d}{dx}\left(x^5+8\right)\\&=\frac{d}{dx}x^5+\frac{d}{dx}8\quad\text{Property of the derivative of a sum}\\&=\frac{d}{dx}x^5+0\quad\text{Property of the derivative of a constant}\\&=5x^{5-1}\quad\text{Property of the derivative of }x^n\\&=5x^4\end{aligned}\]

Therefore, the derivative of \(f’\) is \(f'(x)=5x^4\).

Exercise 4. Find the derivative of the function f defined as: \[f(x)=x^3+5x^2\] Solution: Applying the properties of the derivative, we obtain:

\[\begin{aligned}f’(x)&=\frac{d}{dx}f(x)\\&=\frac{d}{dx}\left(x^3+5x^2\right)\\&=\frac{d}{dx}x^3+\frac{d}{dx}5x^2\quad\text{Property of the derivative of a sum}\\&=\frac{d}{dx}x^3+5\frac{d}{dx}x^2\quad\text{Property of the derivative of a constant multiple}\\&=3x^{3-1}+5\cdot 2x^{2-1}\quad\text{Property of the derivative of }x^n\\&=3x^2+5\cdot 2x\\&=3x^2+10x\end{aligned}\]

Therefore, the derivative of \(f\) is \(f'(x)=3x^2+10x\).

Learn more about the derivative and its properties in: Derivative of A Function- Calculus

Recommended article: Properties of logarithms solved exercises